4.1 Cryptography.
There are a few brands of cryptography that love to play this hide and seek game on the Internet: “I will give you a
semiprime, you go ahead and find the two primes whose product the said semiprime is. If you can”. The mathematicians
concerned, having chosen rather large primes for their game, bet that you could never ever find those two primes and went
ahead and secured the Internet on that assumption. You could prove them wrong, who knows. You just go ahead and try it.
But before continuing, let us agree on some names: let us call S the mathematicians’ semiprime, p1
the smallest of both primes and p2 the largest one.
As we know, S, p1 and p2 are all in A. So, the first thing you have to do
is to convert into a Y-element the semiprime you got from the mathematicians (simply apply integer division by 3 on S).
In the second place you have to take notice of the rest value you get from the division by 3 of the mathematicians’ semiprime.
Since it is never divisible by 3, that rest value will always be either 0.3333... or 0.6666... Let us then agree that we are
going to call S3 the former and S6 the latter, all in accordance with their rest value.
In order to solve the mathematicians’ little game all you need is a table that looks like this:
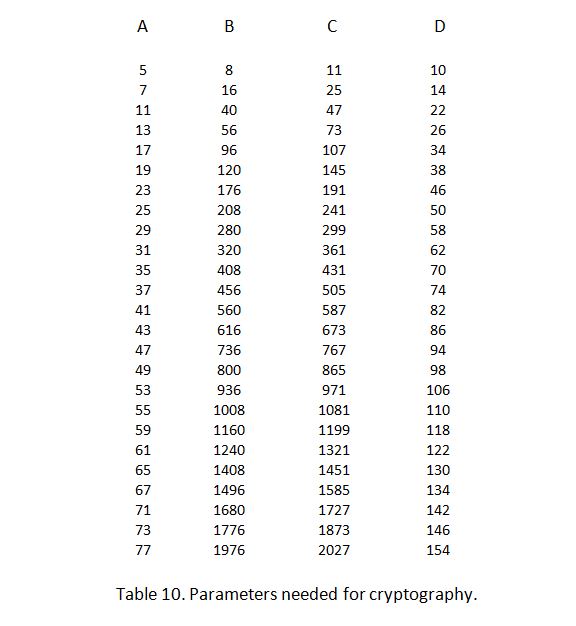
Column 1 of Table 10 is simply A. On column 2 you see the first index of all odd-numbered checking rounds of Table 1;
on column 3 you see the first index of all even-numbered checking rounds, also of Table 1 and finally, on column 4 you see the
checking frequencies that you saw earlier, also on Table 1.
So Table 10 is somehow hybrid in that it contains indexes and frequencies related to Y but it also contains A.
You should be aware of this.
So we are going to suppose you got from the mathematicians a semiprime of the type S3, for instance 555,991.
You convert it to Y-element by dividing it by 3:
S3 = 555991/3 = 185330.3333
The next thing you do is to compute s, where
s = √S
In this case is s:
s = √555991 = 745,64
So S3 is the 185,330th element of Y and s is equal to 745 (in both cases rest values are disregarded.)
At this point we have to introduce a new value, t. By now you should be aware of, that during the checking rounds there
are elements of Y that get checked zero times (the primes) while others get checked one time, two times, three times...
Well, we are going to designate with t the number of times an element of Y gets checked during the whole checking
process. In this context we will be talking of the t-value of a particular element of Y.
Being the square root of the product of two different factors always greater than the smallest of both factors, and having
S a t-value of 1, holds necessarily that p1 is smaller than 745 and p2 greater.
(By the way, if s is not an element of A, you should replace it by the nearest A-element that is greater
than s. In this case it is not necessary as 745 is not divisible by 2 or by 3 and is therefore an A-element).
By now you know that the primes you are looking for are at both sides of 745. You have to take your odds: which one is nearer
to 745, p1 or p2? The nearer it is, the easier it is to find.
There is no way to know and that is why I recommend you go and look for p1. The reason is that the operations
you have to perform next, tend to give a negative result if you look for p2. It really doesn’t matter very much,
but a number that is unexpectedly negative could lead to confusions. But, if you feel comfortable, just go ahead and try to find
p2 instead of p1.
At this point you are ready to perform some operations on Table 10. I actually took this table from Excel and so I have called the
columns A to D. And incidentally, I strongly reccomend that you expand Table 10 in Excel so far as needed for otherwise you
will miss the numerical explanation I will be giving below on how to find p1 and p2.
Before going any further I ought to make a general comment: If you are this far you have to scan Table 10, be it upwards if you
are looking for p1, be it downwards if you are looking for p2. But if you look at all 4 columns
of Table 10 it will be no surprise to you that as you initiate your search of the two prime factors, you could yourself generate all the
data on that table as you go. So, if you are looking for the factors of a large semiprine it could well be that you are better off
if you already have generated and saved such a table in advance. The advantage is that it speeds up your search tremendously but the
disadvantage is that it takes a lot of space to save.
The first step to begin scanning Table 10 is to choose an entry where to begin. Well, that entry is given by s and that is
why you had to convert it to become an A-element if necessary. You can find A in column 1 of Table 10.
So in this case you look for 745 on column 1 and that is the row where your scanning begins. While staying in that row, you compute:
(S3 - B) / D
By “B” and “D” I mean the values that you will find in a spread sheet if you prolong Table 10 downwards. In this case the values found are:
(185330 – 185008) / 1490 = 0.2161
It is extremely important that you subtract B and not C from S3. You subtract C from S6, i.e.
when the rest value we talked about earlier is 0.6666…
The value to keep an eye on is of course the result of the operation, in this case 0.2161. Since your first result is not a whole
number, you have to scan one row upwards on Table 10, and that is the raw that shows 743 on the first column. You repeat the
operation given above, you see that the result is 0.8842, not a whole number and you move ahead, another row upwards. After a while
you arrive at a row that shows 613 on the first column. You then compute:
(185330 – 125256) / 1226 = 49
Since you got now a whole number with no decimals, this is the place to be. You have found p1 on the first entry of this row, so
p1 = 613
And if you divide the mathematicians’ semiprime by p1, you find of course p2:
p2 = 555991 / 613 = 907
It is as easy as that.
4.2 Your odds.
I realise very well that I have arrived at p1 and p2 departing from a table. Such a table
is easily made and saved to factorise small semiprimes. When you are dealing with 150 to 200-digits numbers, making and saving a
table that is suitable to facilitate factorisation of such semiprimes is quite another thing. The fact of the matter is though
that if you apply the present algorithm, you will be able to factorise any semiprime without executing one single trial
division.
Generating primes means dealing with large numbers. By the time you are generating primes as large as 36,000,000 the difference
between the starting point of a checking round and the starting point of the next one is about 24,000. This means that there are
24,000 Y-elements that are being checked before a new checking round begins. Of these 24,000 Y-elements only about
1,512 are primes. This means that the other 22,488 are composites. If you realise that by that time there are 4,000 checking rounds
active and that the Y-elements have an average t-value of 400 (meaning that they get checked an average of 400 times),
then you will have to conclude that the composites that have been checked only once (and these are the mathematicians’ semiprimes)
are a very small percentage of those 24,000. And we are “only” talking of 8-digits primes.
My guess is that as the number of checking rounds keeps growing, there comes a point where the percentage of composites that get
checked only once, begins to decrease. But irrespective of the veracity of this last assertion, if your data base (that is no other
thing than a huge Table 10) is intended to factorise semiprimes, you could consider incorporating into it only what you are looking
for: semiprimes. And you do this by creating a huge Table 10 where you admit only rows whose first entry an A-element is
that gets checked only once during the whole checking exercise (I don’t want to confuse you, but Y gets checked, not
A, so when I was just talking about “an A-element that gets checked” I mean actually its corresponding
Y-element).
Further, you should know that the semiprimes you are bound to look for in your huge Table 10 have 1, 3, 7 or 9 as an end digit.
Due to a super abundance of A-elements with 5 as end digit, the other 4 just mentioned constitute each about 15% of all
A-elements. You could use this to speed up your searching procedures.
Finally, if you are in the business of finding ALL prime factors of ANY composite, a properly checked Y is the place to be.
I am sure that, arrived at this point, you will be able to do it yourself. It is a real poc, you will see.
Epilogue
Now that you have come thus far and indeed, if you have come this far, I wonder who you are. I wonder if you liked it or disliked it, if you learned anything from it or not.
In a way, my readers are my friends. That is what I think when someone reads one of my books. So, if you have read this site, you are one of them.
Anyway, and for what it is worth, I am convinced that our numerical system is unbalanced. No one will ever convince me that the distance between 0 and 1 is equal to the distance between 1 and 2. No one will ever convince me that he has reached comprehension of the number zero. No one will ever convince me of the equidistance that is said to exist within the sequence of the so-called natural numbers. These are the convictions that led me to try to find the prime numbers in a place other than the one where the mathematicians had been looking for them.
I see, as everybody does, that engineers are extremely clever people: they bring us to the moon and back. But I am convinced that Big Science is wrong in claiming for themselves the successes the engineers have achieved. But most of all, I am convinced that Big Science is wrong while trying to measure the universe using the same tools the engineers use to measure the length of an iron bar.
In a few words, engineers are free to place their zero point of measurement any place they want while Big Science, lacking both a ruler to measure and an iron bar to be measured, have absolutely no place where to fix the zero measurement point they claim to possess. Whenever you multiply Mass by anything squared, be it the speed of light, be it the speed of a snail, the results will always be the same: Unknown.
With these considerations I went ahead and tryed to find the prime numbers. Whether I found them or not, you tell me.
After all these reflections, I will end up with the next corollary: there must be an infinite number of primes for if that were not
the case then there should be at least one x for which it holds that x2 ≠ x2.
____________________________________________________________________
Click below to order a book.








.
N/A